HARMONIC PERIODIC SYSTEM AND GENETIC LAWS OF CHEMICAL ELEMENTS

Eng. Julio Antonio Gutiérrez Samanez

 (ABSTRACT)

                                                                                         

The Periodic Law of chemical elements that was first enounced by the russian chemist Dimitri Ivanovich Mendeleiev, in 1869, almost paralelly with the german Lothar Meyer, establishes that the properties of the chemical elements are periodic functions of their atomic weigths. But this law is only a theoretical enouncement not a law expressed in a mathematical function.

 

Based on the work of a great cusquenian scientist, almost forgotten by official science, Dr. Oswaldo Baca Mendoza (1908-1962), we have developed some ideas that try to meet that mathematical expression, that, grouping and periodifying the chemical elements, in function to their atomic numbers (Z) and other quantical parameters, show us what would be the formulas of universal matter. We can synthetize the work as follows:

Dr. Baca Mendoza proposed in his paper “Genetic laws of the chemical elements: New Periodic System”, Cusco 1953, the expression (1) or “Law of successive formation of immediate nucleus” that we call “Law of horizontal and diachronic distribution of elements”:

 

Z = k + [1(n)]   (1), for values  k = 1 and n ³ 0,

being able to define the infinite natural series of the formation of the nuclei of the chemical elements.

We know the main quantical number n represents the floors or levels of energy of the atoms, at the same time each level of energy has sublevels  an orbits defined by the quantical numbers l, ml and ms. The sublevels are denoted by the letters s, p, d, f, g, h, i ... etc, and contain the electrons ordered according the infinite series: 2, 6, 10, 14, 18, 22, 26, 30,... ¥

 

It is a recurrent series that results of the expression:

Number  of electrons per sublevel    = 2 (2n – 1)   to n ³ 1.                                        (2)

One of the orbital orderings we will call “static” is as follows:

 

 

However the natural “dynamic” or harmonic ordering know as “Aufbau” , that shows the resulting “translappings” of the interactions of energy in the sublevel, the increasing of the atomic number and the symmetry effects, is what is seen in the series and following dispositions: (Levels of energy, Notation of the sublevel, Quantical magnetical orbital number and Number of electron per sublevel, respectively).

 

 

 

 

 

 

 

 

 

 

 

 

 

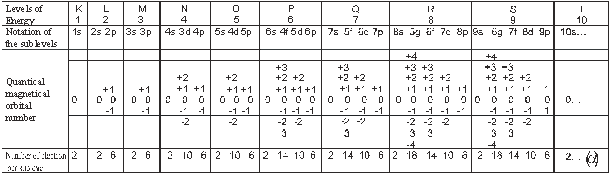
From this infinite series (a),   we obtain the Law of electronic configuration

Electronic configuration =2 (1,1,3,1,3,1,5,3,1,5,3,1,7,5,3,1,7,5,3,1,9,7,5,3,1,9,7,5,3,1.....)  (3).

[where the number of electrons per sublevel increases according to the expression (2)]

From the series   (a), we can deduct two systems of harmonic periodification A and B.

 

PERIODIFICATION SYSTEM A

(2); (2,6); (2,6); (2,10,6), (2,10,6); (2,14,10,6); (2,14,10,6); (2,18,14,10,6); (2,18,14,10,6)....

 2      8        8        18          18             32                32                50                     50 ......       (b)

 

 

 

PERIODIFICATION SYSTEM  B

 

(2); (2);  (6,2); (6,2); (10,6,2), (10,6,2); (14,10,6,2); (14,10,6,2); (18,14,10,6,2); (18,14,10,6,2)....

 2     2       8        8        18          18             32                32                50                     50 ......       (g)

 

Let’s observe that in these series the electronic ordering also corresponds, symmetrically, to the number of electrons existing in each period, therefore, operating with the expression  (b) and (g) we obtain the periodic laws that Baca Mendoza called the genetic laws, however, what I propose in this paper differs from what the researcher proposed.

 

 

PERIODIC LAWS OR OF THE LIMITATION OF PERIODS

 

(PA) FOR SYSTEM A 

2      8        8        18          18             32            32         50            50 ......       (b)

                2   2(2²)   2(2²)   2(3²)     2(3²)          2(4²)        2(4²)       2(5²)     2(5²).....  taking a common factor:

P_A = 2 (1,  2²,  2²,   3², 3²,  4², 4²,  5², 5²..... )                                                       (4)

 

 (PB) FOR SYSTEM   B 

2     2       8        8        18        18        32        32       50        50 ......       (g)

2     2    2(2²)   2(2²)   2(3²)    2(3²)    2(4²)   2(4²)    2(5²)    2(5²).....   

P_B = 2 (1, 1,  2²,  2²,  3², 3²,  4², 4²,  5², 5²..... )                                                    (5)

 

 

These Laws mathematically define the periodifications. In the system A [series (b)], after the first term, 2 (Block of elements from the first period), the periods are paired or binodic (the expression binode was introduced by Dr. Baca): 2, 8 ,8, 18, 18, 32, 32, 50, 50, 72, 72, 98, 98,... and, in the system B, all  the periods are paired or binodic, therefore, they have an exact symmetry in their increasing  (2,2, 8, 8, 18,18, 32, 32, 50,50,...) ó (4, 16, 36, 64, 100, 144, 196....in binodes). This binodic serie B, reduces it self to the general parabolic expression or second grade function Y = 4m². where Y is the growing up periodic function of m, (m≥1), whish is number the couple of periods of binode.

Adding up the terms in the series we have Z = 4 ∑(m_i)² ; for i=1 to n, and Z (atomic number)

 

With all this we can design the periodic charts for both systems:

               

 

 

 

We can distribute these ordered series by levels of energy in two systems:

 

 

	SYSTEM A		SYSTEM B
Form A-1	Form A-2		Form B-1	Form B-2
1s	1s		1s	1s
2s 2p	2s 2p		2s	2s
3s 3p	3s 3p		2p 3s	2p 3s
4s 3d 4p	4s 3d 4p		3p 4s	3p 4s
5s 4d 5p	5s 4d 5p		3d 4p 5s	3d 4p 5s
6s 4f 5d 6p	6s 4f 5d 6p		4d 5p 6s	4d 5p 6s
7s 5f 6d 7p	7s 5f 6d 7p		4f 5d 6p 7s	4f 5d 6p 7s
8s 5g 6f 7d 8p	8s 5g 6f 7d 8p		5f 6d 7p 8s	5f 6d 7p 8s
9s 6g 7f 8d 9p	9s 6g 7f 8d 9p		5g 6f 7d 8p 9s	5g 6f 7d 8p 9s
			6g 7f 8d 9p 10s	6g 7f 8d 9p10s

 

       

 

The same distribution expressed in terms of maximum number of differentiating electrons per level and sublevel, also corresponds to the number of elements per levels of energy and blocks  (s, p, d, f,..)

 

	SYSTEM A		SYSTEM B
Form A-1	Form A-2		Form B-1	Form B-2
2	2		2	2
2 6	2 6		2	2
2 6	2 6		2 6	2 6
2 10 6	2 10 6		2 6	2 6
2 10 6	2 10 6		2 10 6	2 10 6
2 14 10 6	2 14 10 6		2 10 6	2 10 6
2 14 10 6	2 14 10 6		2 14 10 6	2 14 10 6
2 18 14 10 6	2 18 14 10 6		2 14 10 6	2 14 10 6
2 18 14 10 6	2 18 14 10 6		2 18 14 10 6	2 18 14 10 6
			2 18 14 10 6	2 18 14 10 6

The reader would observe that the increasing number of blocks of elements is a function of the increasing number of electrons in the levels and sublevels. (s, p, d, f, g)

 

LAWS OF VERTICAL GROUPING OR OF GROUPS

This periodicity is functional and harmonic, with the Law of vertical grouping or synchronic of elements(Zg) that the Dr. Baca called Law of groups  that is a result of adding up the terms in each one of the series  (b) and (g)

FOR SYSTEM A

Operating with the expression (b)

2   +   8     +     8   +     18    +    18     +      32    +      32   +    50     +     50 ......      

2  +  2(2²) +  2(2²) + 2(3²)  +  2(3²)   +     2(4²)  +     2(4²)  +  2(5²)  +  2(5²).....    Taking a common factor:

2 (1 + 2²  +  2² + 3² +  3² +  4² +  4² +  5² +  5²  +..... )  

For the resulting series to start with the unit (Hydrogen, 1H),  we introduce zero in the sum and add an integer Z ³ 1 to the whole expression, to get:

ZgA = Z + 2 ( 0 + 1 + 2²  +  2² + 3² +  3² +  4² +  4² +  5² +  5²  +..... )                   (6)         

 For example for Z=1

 ZgA = 1, 3, 11, 19, 37, 55, 87,....   That corresponds in the chart to the vertical group :

         = 1H, 3Li, 11Na, 19K, 37Rb, 55Cs, 87Fr,.....

 

FOR SYSTEM B

Operating with the expression (g)

2  +  2   +   8     +     8   +     18    +    18     +      32    +      32   +    50     +     50 ......     

2  +  2  +  2(2²) +  2(2²) + 2(3²)  +  2(3²)   +     2(4²)  +     2(4²)  +  2(5²)  +  2(5²).....    Taking a common factor:

2 (1 + 1  + 2²  +  2² + 3² +  3² +  4² +  4² +  5² +  5²  +..... )  

Like in the previous case, for the resulting series to start with the unit, we introduce zero in the sum and add an integer  Z ³ 1 to whole expression, which results in:

ZgB = Z + 2 ( 0 + 1 + 1 + 2²  +  2² + 3² +  3² +  4² +  4² +  5² +  5²  +........)                           (7)

To Z=1

 ZgB= 1, 3, 5, 13, 21, 39, 57, 89, ....

        = 1H, 3Li, 5B, 13Al, 21Sc, 39Y, 57La, 89Ac,.....

 

From the interaction of these laws the harmonic systems or periodic charts are constructed, like it is shown below, each one has its ow variations. (Appendix 2.1 and 8.3)

 

Summarizing, these laws show the universal validity of dialectics, by  demonstrating that quantitative changes in growing lead to qualitative changes or leaps from an inferior to a superior level, in this case symetrical, paired and exact. This increasing follows the interior dynamics of the atom, always from inside towards outside, both in nuclear increasing as well as electronic

 

SPIRAL ORDERING OF THE PERIODIC  SERIES OF ELEMENTS

 

FIRST LEVEL OR PERIOD (appendix 3.1, red colored curve)

 

  _; 

A polar spiral is drawn from  0 a 2 π, which radium is a function of φ , and takes the value of 1(for hydrogen) for the angle π  or de 180 degrees and the  value of  2 for the angle 2 π,  (360 degrees)

φ	0	π/4	π/2	π	3 π/2	2 π
R1	0	0.25	0.5	1	1.5	2
Elements				H		He

 

 

 

 

 

 

 

 

SECOND LEVEL (Appendix 3.1, orange colored curve)

It is a spiral that starts in the radium 2, like origin, and goes from  0 a 360 degrees or 2π dividing  in eight sections  the circle in which it inscribes, corresponding to eight elements from  3LI al 10 Ne. According to the formula: R = 4/π φ;    R₂ = R+2

φ	π/4	π/2	3π/4	π	5π/4	3π/2	7π/4	2 π
R	1	2	3	4	5	6	7	8
R2 = R+2	3	4	5	6	7	8	9	10
Elements	Li	Be	B	C	N	O	F	Ne

 ; 

 

 

 

 

 

 

THIRD LEVEL (Appendix 3.1, yellow colored curve)

Spiral that stars in the radium  10 at 0 degrees and goes on, dividing the circle in witch it inscribes in eight sections , like in the previous case, to the radium 18Ar. According to the formula: R = 4/π φ;    R₃ = R+10

                              ;                             

 

φ	π/4	π/2	3π/4	π	5π/4	3π/2	7π/4	2π
R	1	2	3	4	5	6	7	8
R3=R+10	11	12	13	14	15	16	17	18
Element	Na	Mg	Al	Si	P	S	Cl	Ar

 

FOURTH LEVEL (Appendix 3.2, green colored curve)

The spiral starts in the radium 18Ar and goes to the radium 36Kr, in the angle φ= 2π, in a circle divided in  18 sections, according to the formula: R = 9/π φ;    R₄ = R+18

               ;              

 

 

 

FIFTH  LEVEL (Appendix 3.2, Blue colored curve)

The spiral starts in the radium 36Kr. and goes on, again inscribed in a circle of 18 sections to the radium 54Xe,  in the angle 2π. According to the expression : R = 9/π φ;    R₅ = R+36

                              ;                             

 

 

SIXTH LEVEL (Appendix 3..3, indigo colored curve)

The spiral starts en radium  54Xe, and goes on  now inscribed in a circle of 32 sections, to the radium 86Rn. In the angle 2π. According to the expression:

R = 16/π φ;    R₆ = R+54

 

                              ;                

 

 

 

 

SEVENTH LEVEL (Appendix 3.3, violet colored curve)

The spiral starts in radium 86Rn , and goes inscribed in a circle also divides in 32 sections, to the radio 118 Dsc (unknown rare gas) in the angle 2π. The formula is :      R = 16/π φ;    R₇ = R+86

                              ;                             

 

 

 

* Dsc :     Unknown

** Hypothetical rare gas Peruvion: honoring the country of the author

 

The levels or periods eighth and ninth will have spirals inscribes in circles of 50 sections,          (2 x 5x5)

 

           ;                             

 

The levels tenth and eleventh will have spirals  inscribed in circles of 72 sections..

 

           ;                             

 

The periods twelfth and thirteenth will have spiral inscribed in circles of 98 sections

 

           ;                             

 

Summarizing the general relation for system A, this will be :

R = R1, R2, R3, R4, R5, R6, R7,  ……

 

R = [ 1/π j];[4/π j+2];[4/π j+10];[ 9/π  j+18];[9/π j +36];[16/π j + 54];[16/π j + 86]….

 

R=[1/π j];[2²/π j +2];[2²/π j +10];[3²/π j+18];[3²/π j+36];[4²/π j+ 54];[4²/π  j+ 86]….

 

For system B the mathematical relation will be :

R =[1/π j]; [1/π j+2]; [2²/π j +4];[2²/π j +12];[3²/π j+20];[3²/π j+38];[4²/π j+ 56];[4²/π  j+ 88]….

 

The following graphics are appendix for system A.

 

This is how we summarize our book: "Harmonic Periodic System and Genetic Laws of Chemical Elements", to honor the memory of Dr. Oswaldo Baca Mendoza, in whose creative work we were inspired, developing, improving and overcoming it dialectically like the master would have liked, after half century of conspiration of forgetfulness, silence and incapability of assimilation by official science. In the book we expose with the corresponding detail in each one of the topics, form more information, the reader can access our  web page and to furthermore communicate your opinions and comments to the e-mails

Some "rough drafts" of the book have already been forwarded to colleagues and prestigious scientist aiming for their authorized comments.

                                                                                          Cusco, May 9th,  2003

 

Eng. Julio Antonio Gutiérrez Samanez

Consultant in Ceramics Technology specialized in Japan and professor in SENATI

www.harras.be/hvar/kutiry ; kutiry@mixmail.com ; kutiry@hotmail.com

Calle Inca 357 Santiago Cuzco Perú

 

(El autor agradece la traducción realizada por los ingenieros: Orestes Villafuerte Romero y Juan Carlos Villafuerte Medina)